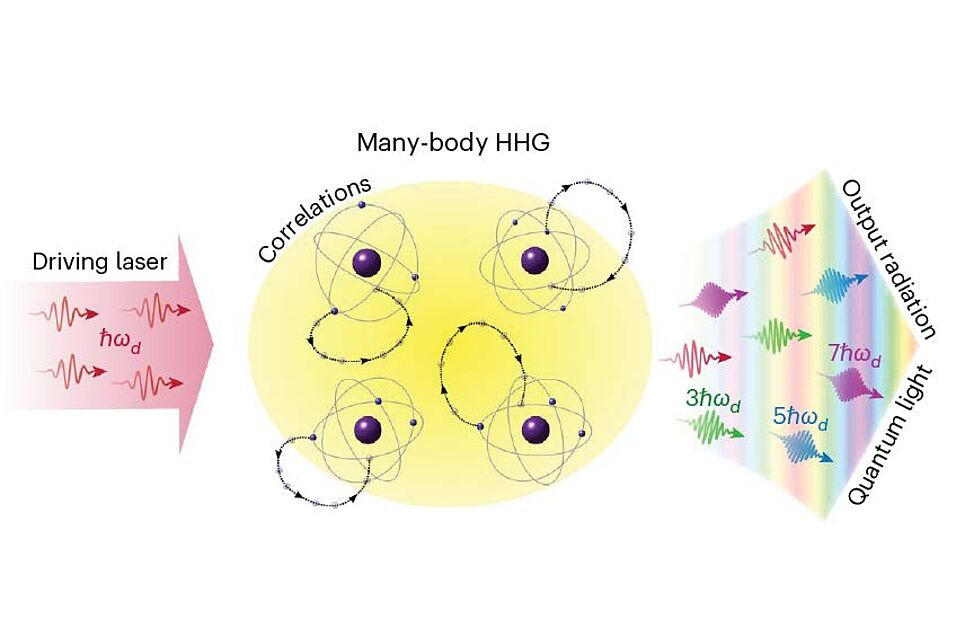
Atoms and molecules subject to a strong laser can emit high-frequency light. This process is called high harmonic generation (HHG) and can to first approximation be understood in a three-step picture: (i) the strong driving laser ionizes some of the particles, (ii) the free electrons continue to absorb photons from the drive and gather more and more energy, (iii) some of these free electrons eventually recombine with the ions, releasing their excess energy in the form of a high-frequency photon. The resulting emission spectrum can contain up to several hundred harmonics whose frequencies are multiples of that of the drive and reach the X-ray spectrum. HHG is an established field of research and considered an attractive source of short high-energy light pulses. A key limitation is, however, that the harmonics are usually in a coherent state of light, offering little flexibility for shaping the quantum state of the light, which would be very desirable for many applications in quantum optics, quantum information, and spectroscopy.
Writing in Nature Physics, the authors theoretically predict that strongly non-coherent states of the harmonics can arise in HHG if the emitters are in a correlated quantum state. Building a theory that describes a many-body system of emitters subject to a strong driving laser, the researchers show that a correlated quantum state of the emitters can be transferred to the state of the emitted light, featuring, e.g., super-Poissonian photon statistics, non-Gaussian Wigner functions, and intra-harmonic correlations. The authors discuss two scenarios particularly relevant for experiments, in which the correlations among the emitters are due to spin squeezing and superradiance, respectively. The concept of many-body HHG potentially opens up a novel platform for the generation of light with engineered quantum features and suggests HHG as a tool to diagnose correlations in a many-body system with attosecond time resolution.
Publication in Nature Physics
Light emission from strongly driven many-body systems
Pizzi, A., Gorlach, A., Rivera, N., Nunnenkamp, A., and Kaminer, I.
Nature Physics (2023).
https://doi.org/10.1038/s41567-022-01910-7